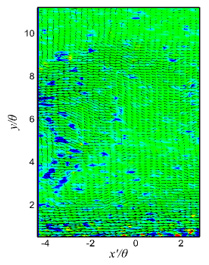
 |
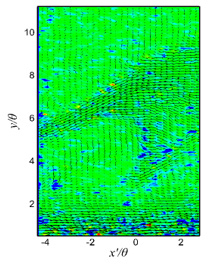 |
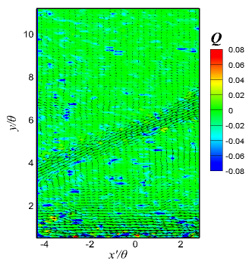 |
| (a) t=t0+0s | (b) t=t0+0.1s | (c) t=t0+0.2s |
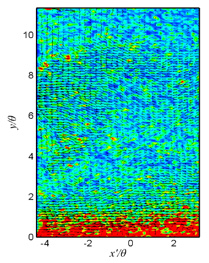
| Figure 4 Contours of second invariant of velocity gradient tensor and fluctuation velocity vectors on x-y plane in active state of drag-reducing flow at x=2400mm |
||
Newsletter 2020.3 Index
Theme : "The Conference of Fluid Engineering Division (March issue)”
|
Study of identification of large-scale turbulence structures in drag-reducing turbulent boundary layer flow by means of stereoscopic PIV measurements
Makoto HIRANO,
|
Abstract
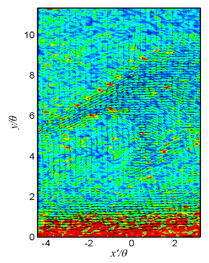
We performed stereoscopic PIV measurements for the drag-reducing turbulent boundary layer flows by injecting surfactant aqueous solution. For the case of the drag reduction ratio more than 60%, large-scale turbulence structures were often observed in the fluctuating velocity field. In this study, we discussed the identification of such large-scale turbulence structures by using the second invariant of the velocity gradient tensor and the imaginary part of the eigenvalues. We used a circulating water tank as experimental device. The circulating water tank has Acrylic test section. An acrylic test plate with a total length of 2796 mm, a width of 387 mm, and a thickness of 20 mm is installed perpendicular to the bottom of the test section. First we checked the reliability of stereoscopic PIV measurement. As can be seen from statics, the stereoscopic PIV measurement was sufficiently reliable except for near the wall. Therefore we judged that we can consider the instantaneous turbulence structures. Next from the instantaneous flow field colored by second invariant of velocity gradient tensor and the instantaneous flow field colored by imaginary part of the eigenvalues, we considered the large-scale turbulence structures. Also the vector represents the velocity fluctuation. Looking at instantaneous flow field, large value of second invariant of velocity gradient tensor is close to large-scale turbulence structures that can be judged from velocity fluctuation. But there is no clear correspondence between large-scale turbulence structures and second invariant of velocity gradient tensor. And instantaneous flow field of imaginary part of the eigenvalues is same. Therefore we revealed the large-scale turbulence structures is structure without strong turning.
Key words
Surfactant, Stereoscopic PIV measurement, Identification of turbulence structures, Drag reduction
Figures

Figure 1 Schematic of experimental equipment
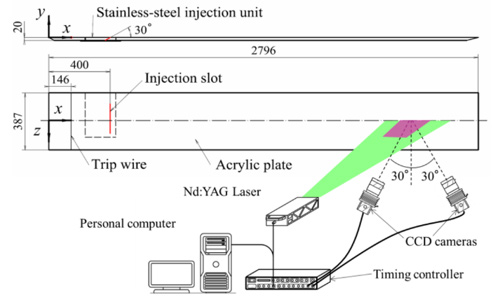
Figure 2 Test plate and stereoscopic PIV measurement system
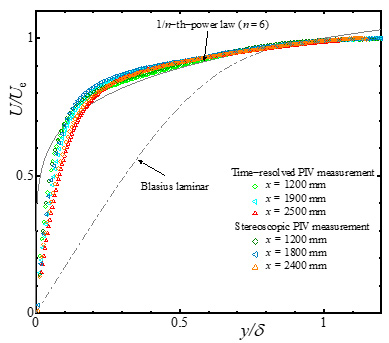
(a)Mean velocity
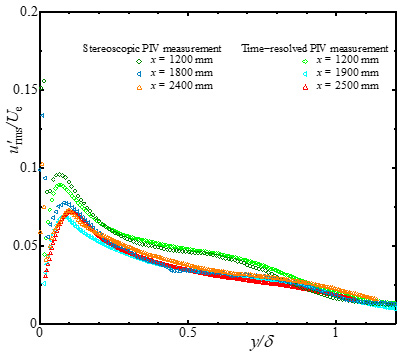
(b)Streamwise turbulence intensity
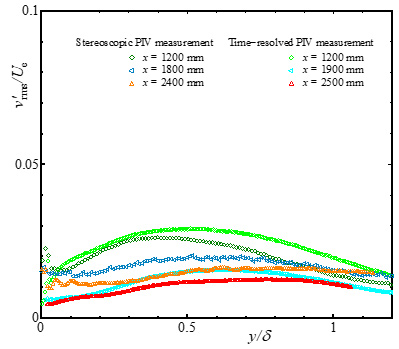
(c)Wall-normal turbulence intensity
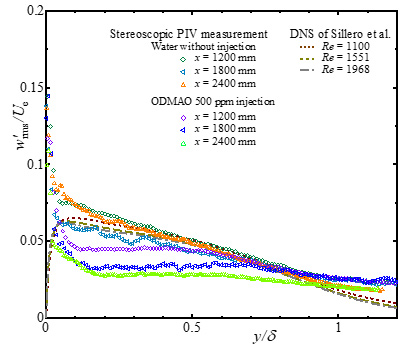
(d)Spanwise turbulence intensity
Figure 3 Profiles of mean velocity and turbulence intensities with outer scaling in drag-reducing flow
 |
 |
 |
| (a) t=t0+0s | (b) t=t0+0.1s | (c) t=t0+0.2s |
| Figure 5 Contours of magnitude of imaginary part of eigenvalue and fluctuation velocity vectors on x-y plan in drag-reducing flow at x=2400mm |
||


