Newsletter  2023.2  Index

Theme : "The Conference of Fluid Engineering Division (February issue)” Preface （T. Hashimoto，S. Matsuda，H.J. Park） Restoration of Cultural Monuments from the Kumamoto Earthquake – Damage, Repair and New Discovery of Cultural Values of Kumamoto Castle, and Brick Buildings of Kumamoto University Ryuichi ITO (Kumamoto University) Numerical simulation of multiphase turbulent flows based on unified volume-average equations Takeo KAJISHIMA (Shikoku Polytechnic College) Large-Scale DES Analysis of Rotor-Stator Interaction Field in a Transonic Axial Compressor Ryugo OZAKI (Kyushu University), Yuki YACHI (Kyushu University) Masato FURUKAWA (Kyushu University), Toshimasa MIURA (Kawasaki Heavy Industries) Development of Innovative PEDOT Synthesis Using Plasma Enveloped Bubble Kazuhiko OHTAKE (Tohoku University), Hidemasa TAKANA (Tohoku University) Modulation of wall turbulence by addition of solid particles Yutaro MOTOORI (Osaka University), Susumu GOTO (Osaka University)

 

Modulation of wall turbulence by addition of solid particles

Yutaro MOTOORI Osaka University Susumu GOTO Osaka University

Abstract

We explore the modulation phenomena of wall turbulence due to the addition of solid particles. For this purpose, we conduct direct numerical simulations (DNS) of finite-size spherical rigid particles in turbulent channel flow by using an immersed boundary method that takes into account fluid-particle interactions. We show the results of DNS with systematically changing the diameter and Stokes number of particles in the turbulence at the three friction Reynolds numbers 128, 256, and 512: We investigate 47 cases in total. Movies of particles in turbulence demonstrate that, when the Stokes number is large enough for the particles not to follow the mean flow well, vortex rings are created around the particles due to the sufficient velocity difference between the particles and fluid. The additional energy dissipation around particles can limit the energy transfer from the mean flow to streamwise coherent vortices; as a result, the total turbulent kinetic energy of the system contained almost by these coherent vortices gets reduced. In other words, turbulence attenuation occurs due to the mechanism that the particles dissipate turbulent energy that would be transferred from the mean shear to coherent vortices. On the basis of this mechanism, we also propose a formula describing the degree of turbulence attenuation.

Key words

Solid particles, Wall turbulence, Vortices, Turbulence modulation

Figures

Movie 1 Visualization in the case with ,  and . Red objects show vortices identified by using the positive isosurfaces of the second invariant of the velocity gradient tensor. White balls are particles.

Movie 2 Visualization in the case with , and . Red objects show vortices identified by using the positive isosurfaces of the second invariant of the velocity gradient tensor. The threshold value is the same as in Movie 1. White balls are particles. We see that compared with Movie 1, coherent vortices near the wall diminish, and instead, ring-like vortices are shedding around particles.

Figure 1 Turbulence attenuation rate. We show the mean of the turbulent kinetic energy of the system in the 47 cases with the addition of particles, normalized by the value for the single-phase flow. Circles, triangles and squares are the results at ,  and , respectively. Different colours denote different .

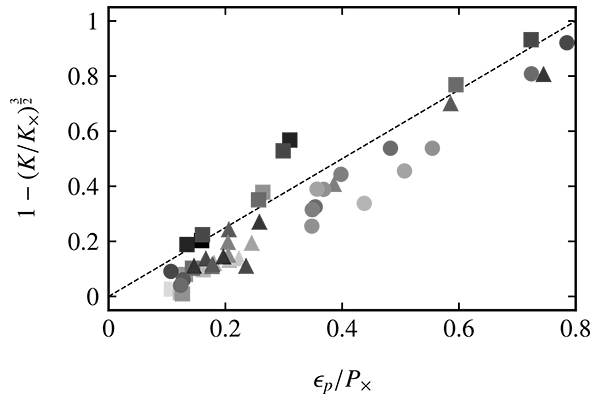
Figure 2 Numerical verification of the formula  describing the attenuation rate of turbulent energy. Symbols are the same as in Figure 1. Dotted line shows . The formula  holds irrespective of the kinds of particles and the Reynolds number.